“Lattice-Filling Curves, Prime Locations, Eulerian Circuits, and the Traveling Salesperson Problem”
Presentation at Bay Area Artists and Mathematicians (BAAM) meeting at Santa Clara University on 2/15/20
I’ve known about prime locations along Ulam’s Spiral in the xy-plane grid since I was young, but I’ve been wondering about a lattice-filling curve in three dimensions for a while now.
Starting with cubical shells, I extended the spiral idea (top) into a helix down the sides of a cube, and spiraling into the bottom (south pole). The first picture shows the primes in green along this path (orange). The next shell out can be covered by a similar path from bottom to top, and in this way, fill space.
Ulam’s Spiral extended along the sides and bottom of a cube
The same can be done with octahedra, arranging concentric shells |x| + |y| + |z| = n and winding from top to bottom, then bottom to top, or one triangular face at a time, as I show in the second picture:
Space can be filled with concentric octahedral shells. Each shell has lattice points that can be visited by a single route, and the path can then jump out to the next shell.
Now the octahedron’s edges can be traced once each by an Eulerian circuit (L), but the cube’s cannot (R), as seen from my pieces at Bridges and JMM. The octahedral path can then be modified to cover all lattice points near the edges, and it comes back to its starting point as well.
Eulerian String Figure Octahedron (L) and Sloppy Fourier Cube (R)
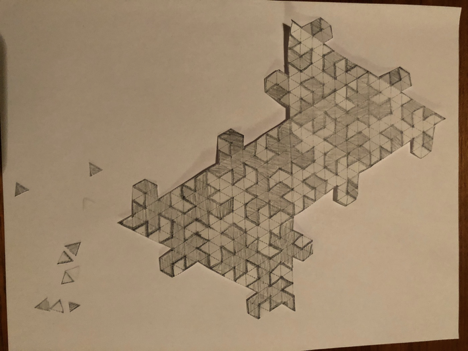
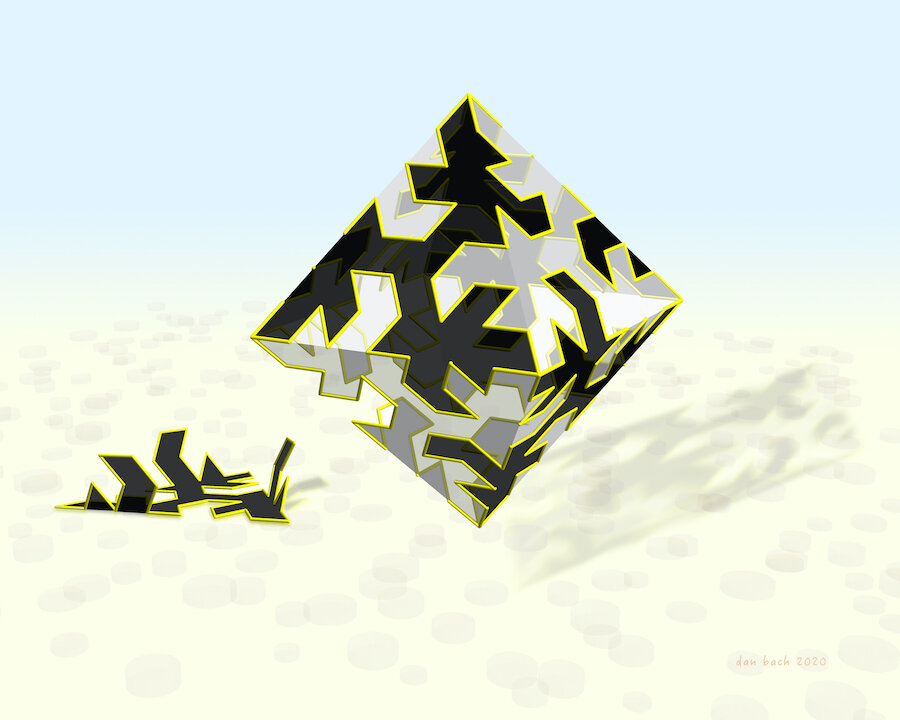
I passed out handouts so people could cut this shape out for the n=7 octahedral layer. It had the added surprising property that each face features the same zig-zaggy polygon in either black or white. This then folds up into the “Zigzag Octahedron” (last picture).
Net for tiled octahedron shown below, copies passed out to the audience!
“Zig-Zag Octahedron” - My entry for the Joint Mathematics Meetings 2021 (Virtual) Math Art Exhibition